A Woven Klein Quartic
Curvature is determined by local geometry, and this can be controlled. A pattern with a particular symmetry is often in an infinite family of patterns, all with the same underlying motif, but of varied curvature
Category: symmetries of things
Symmetry here and there
All of these photos were taken in 2022, for the upcoming The Magic Theorem of the Symmetries of Things, a second edition of the first part of the book, with expanded exercises and examples, in
Various crystallographic space groups
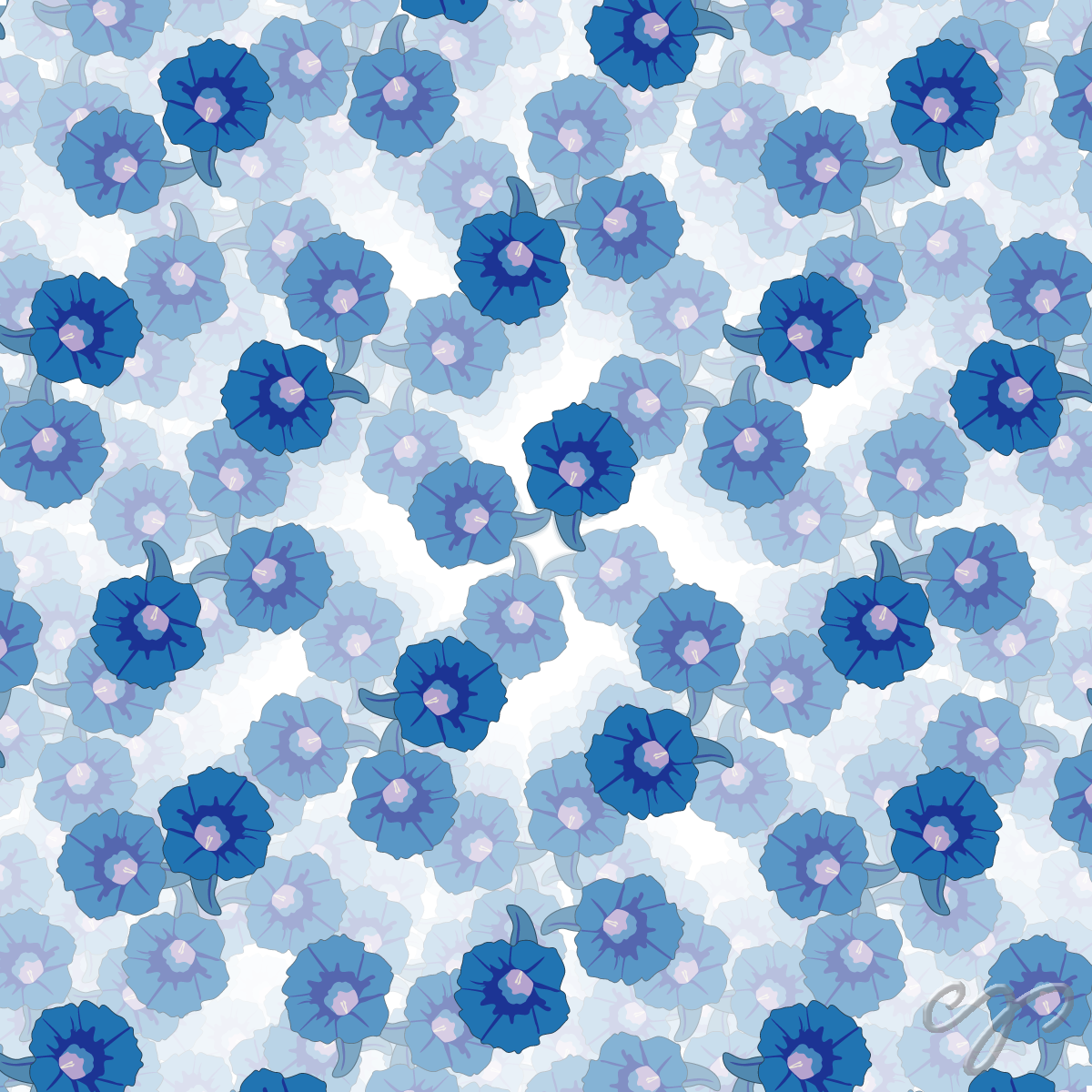
Only a few of these illustrations made it into The Symmetries of Things, but there aren’t such constraints here, and so I present a whole lot of pictures of the non-isotopic, or “composite’ crystallographic symmetries.
Pictures of the kids when they were little
Needless to say, these didn’t make it into the Symmetries of Things. The first pair were rendered in Tess, a program by Chris Watley in 1992 for the NeXT computer. The latter images are seamless
some of the hyperbolic plane illustrations from the Symmetries of Things
These illustrations are from the first edition of The Symmetries of Things, written with John Conway and Heidi Burgiel. Back in the day, kids, I didn’t have a way to actually draw in the computer
Kaleidoscopes
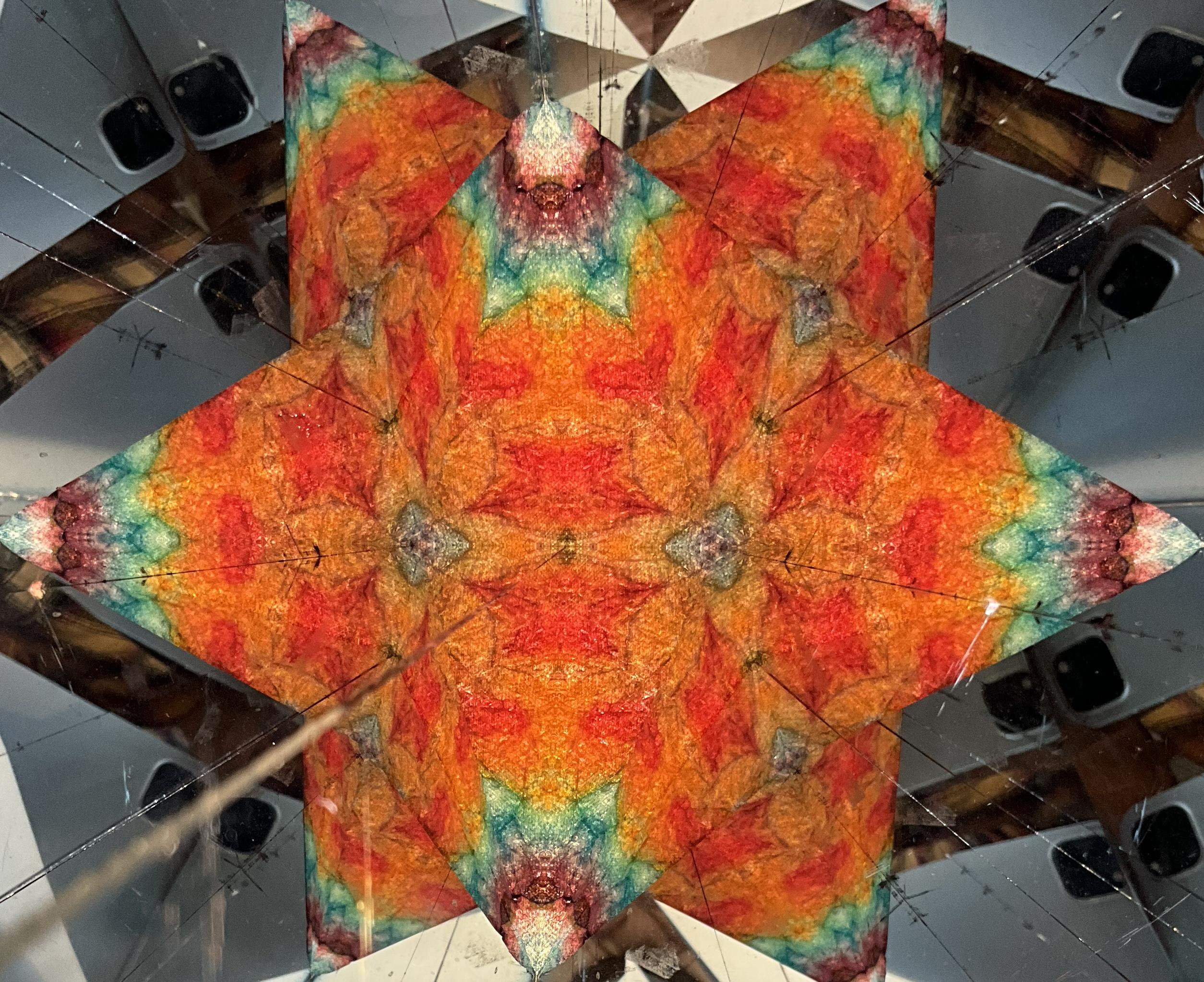
Lots of great new symmetry stuff in the forthcoming Symmetries of Things: The Magic Theorem