Stereopix
You’ll have to cross your eyes or use a stereoscope to see these stereoscopic images, photographed with a stereopi .
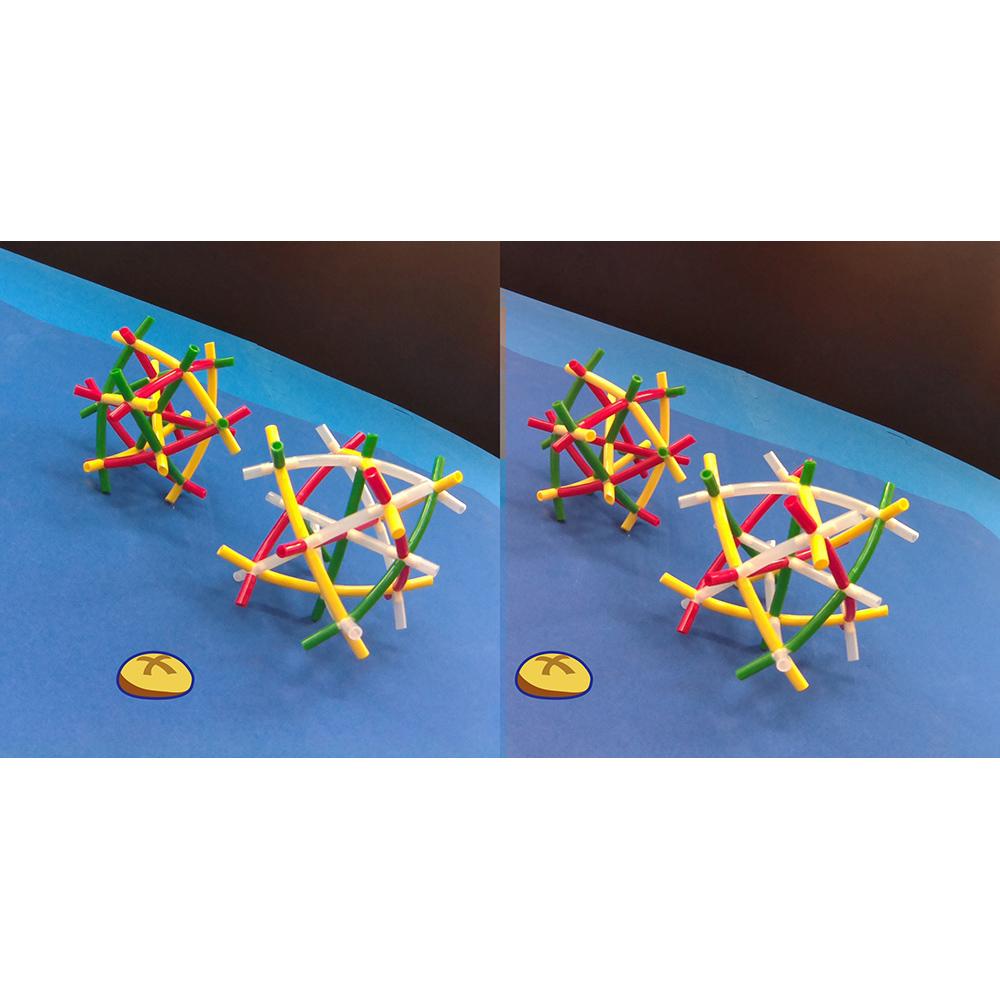
Tubing and 3D printed connecters are a great way to make models with lots of circles, and perfect for stereoscopic images of great circles in the hypersphere. Here are illustrations of a few of discrete symmetries of the hypersphere (Tables 26.1, 26.2 and 26.3 of the Symmetries of Things) — I have many more of these models that I need to photograph. Really though, they would like to be 14′ tall in powder coated aluminum!
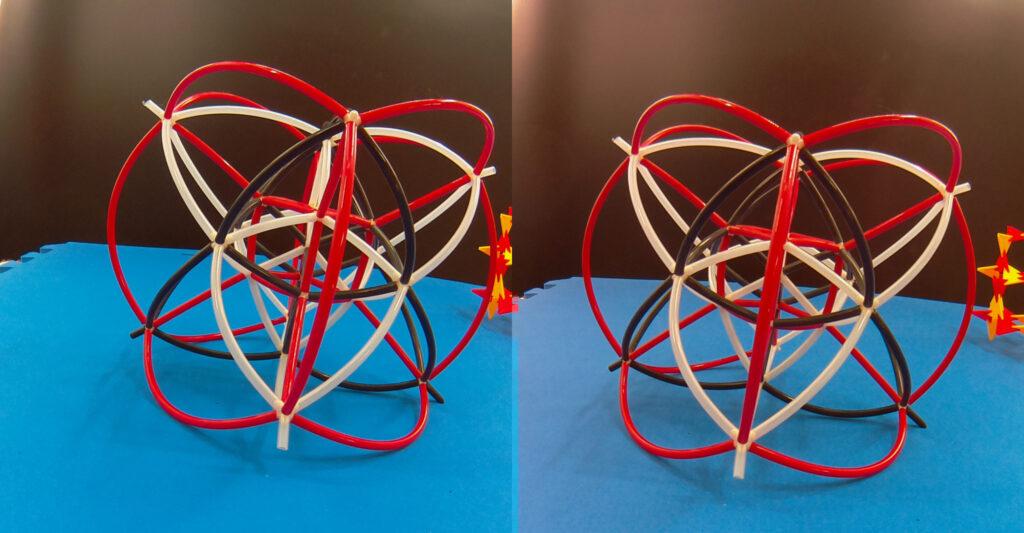
The compound of three hypercubes, or a coloring of the edges of the 24-cell, with symmetry ±1/6 [OxO].
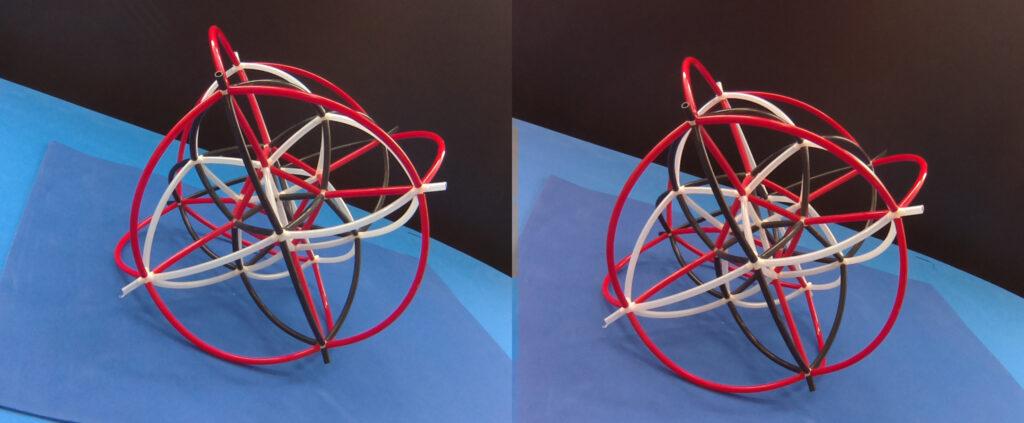
Ultimately, to fully illustrate these symmetries, the plan/dream is to make larger scale sculptures using programmable LEDs within wider diameter tubing. A test piece hung on our porch for about a year, with dynamic glowing colors illustrating subgroups of ±[TxT] (as per the tables). Meanwhile, here are two more subgroups of the more complicated ±[OxO] — the wiring defeated my LED ambitions for the time being.
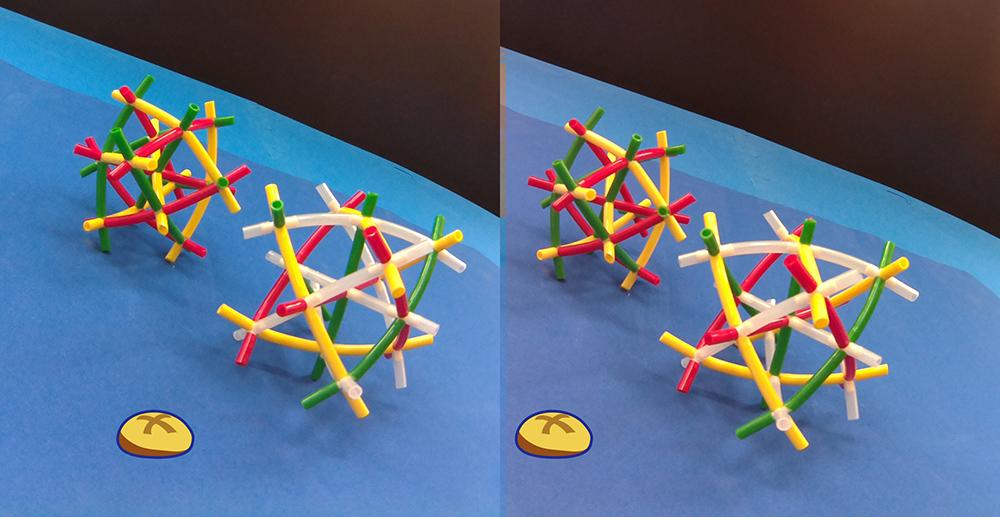
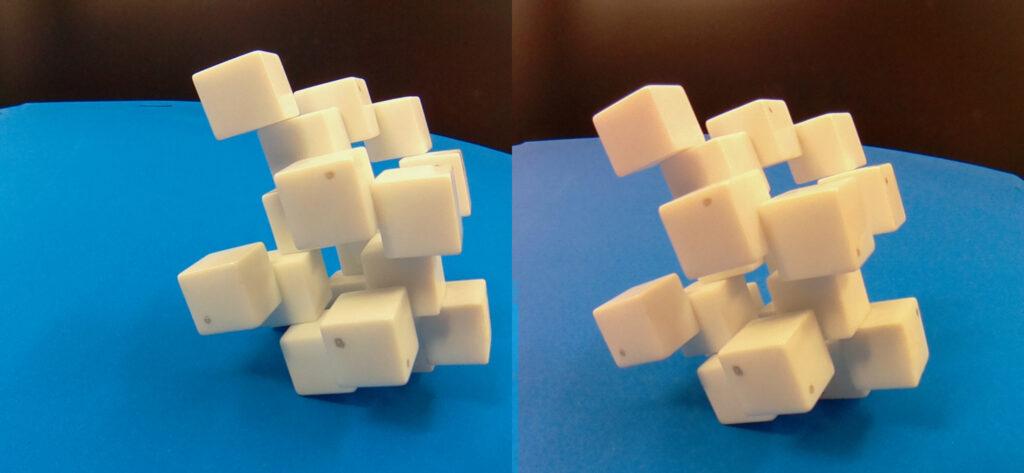
In the background is a model of made of cubes, that can be extended to fill all space, using a simple rule — the cubes each meet four others, gyrorotationally (with local symmetry 2X). The infinite model would have the mysterious quarter group symmetry, 8o:2 , #229 Intl No. The quarter groups, all sub symmetries of this one, are not easy to visualize, and there will be a post just on the various models and sculptures I’ve produced to illustrate these as best I can. Many of those were suggested by Conway, who himself was fascinated and beguiled by these strange space symmetries.
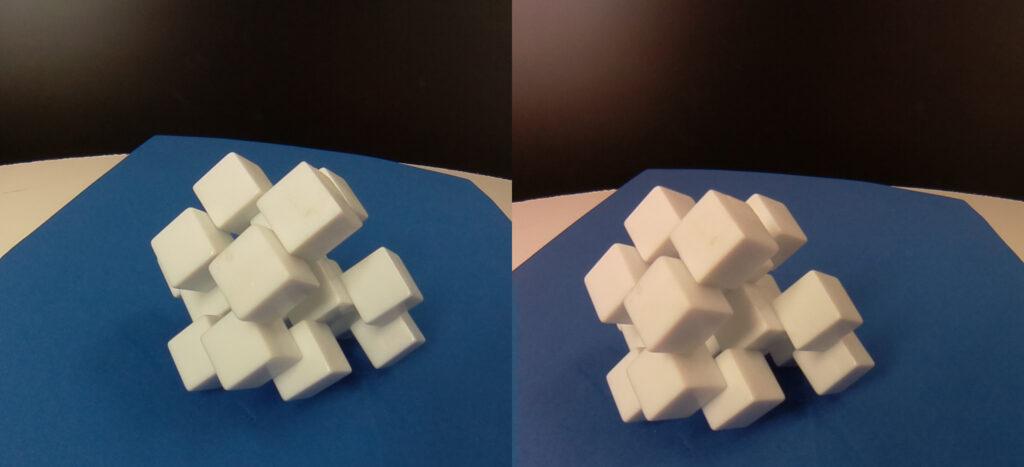
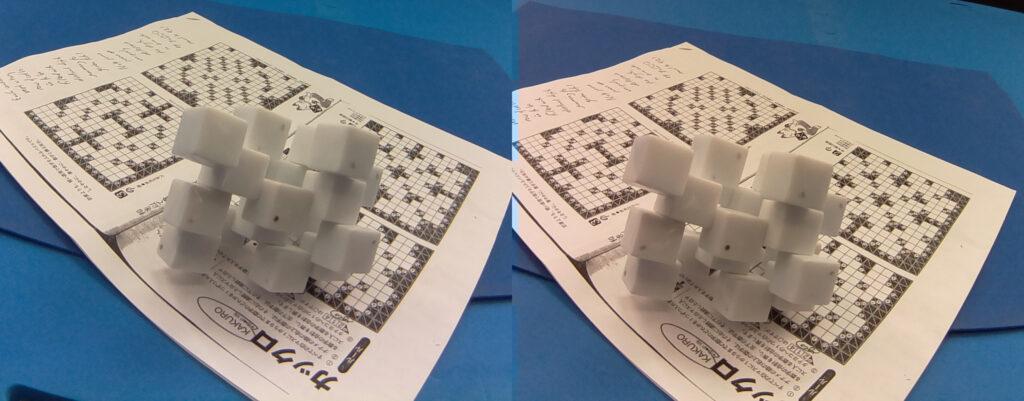
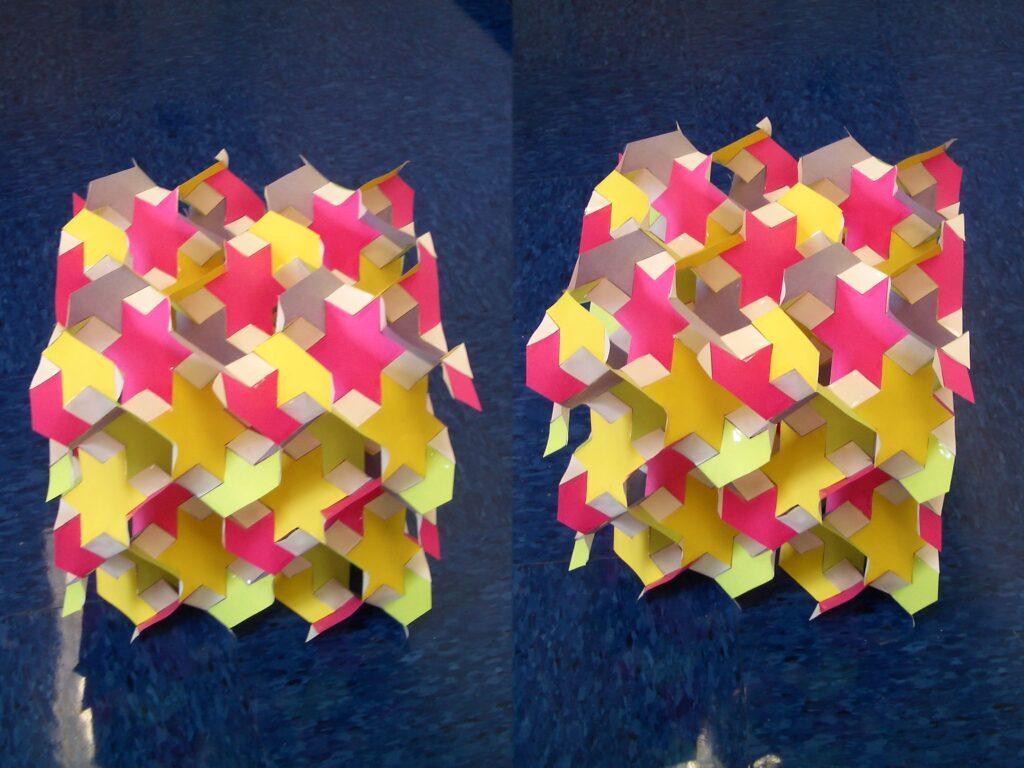
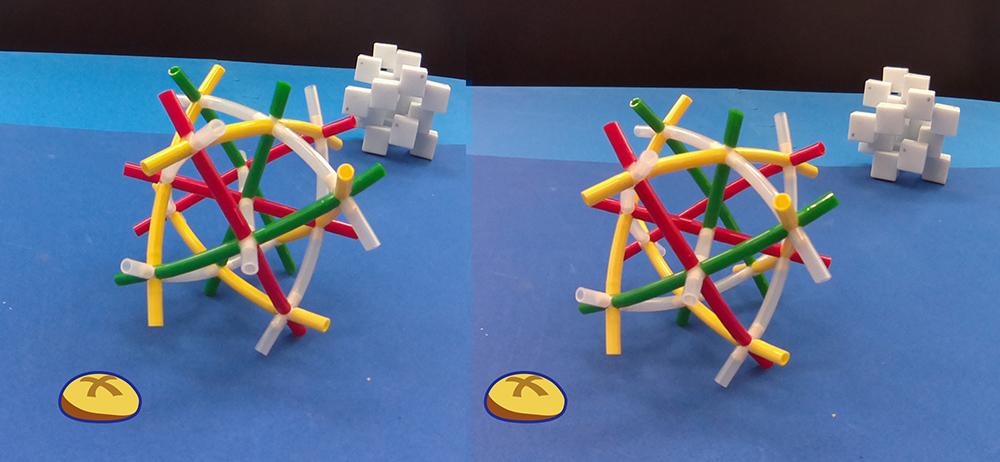
Here is another interesting quarter group symmetry, one of many possible using this system of injection molded pieces, forming a coloring of the triamond or Laves lattice. This system will show up in another post, but these are the only stereoscopic pix I took.
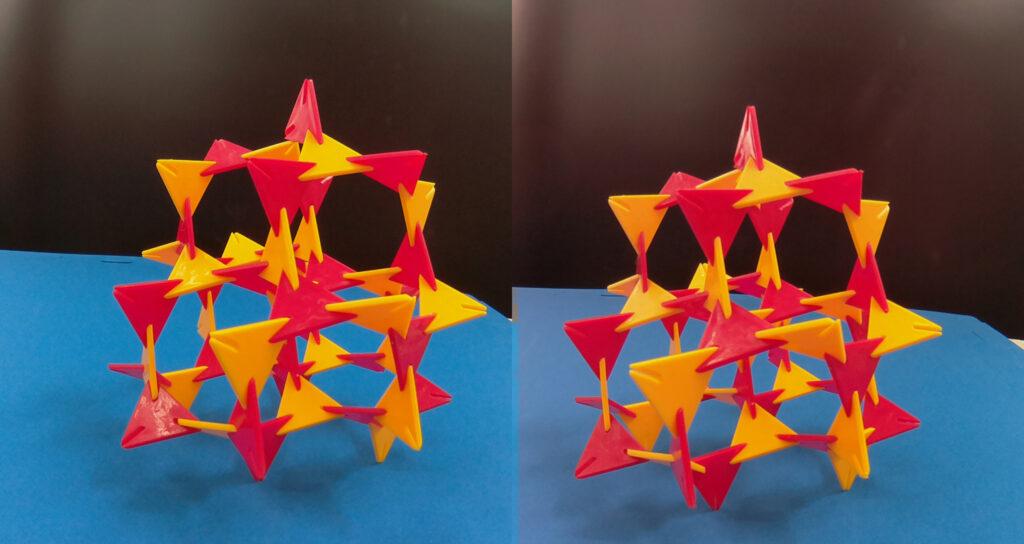
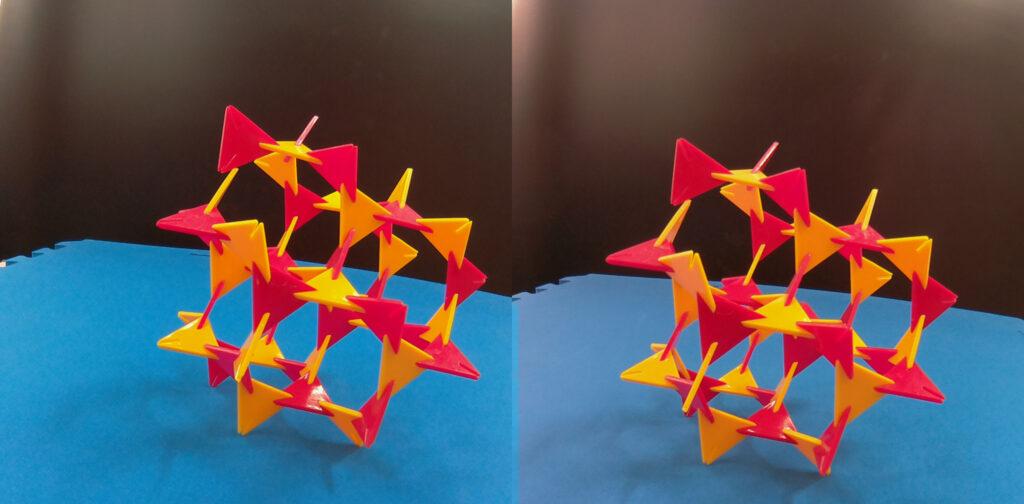
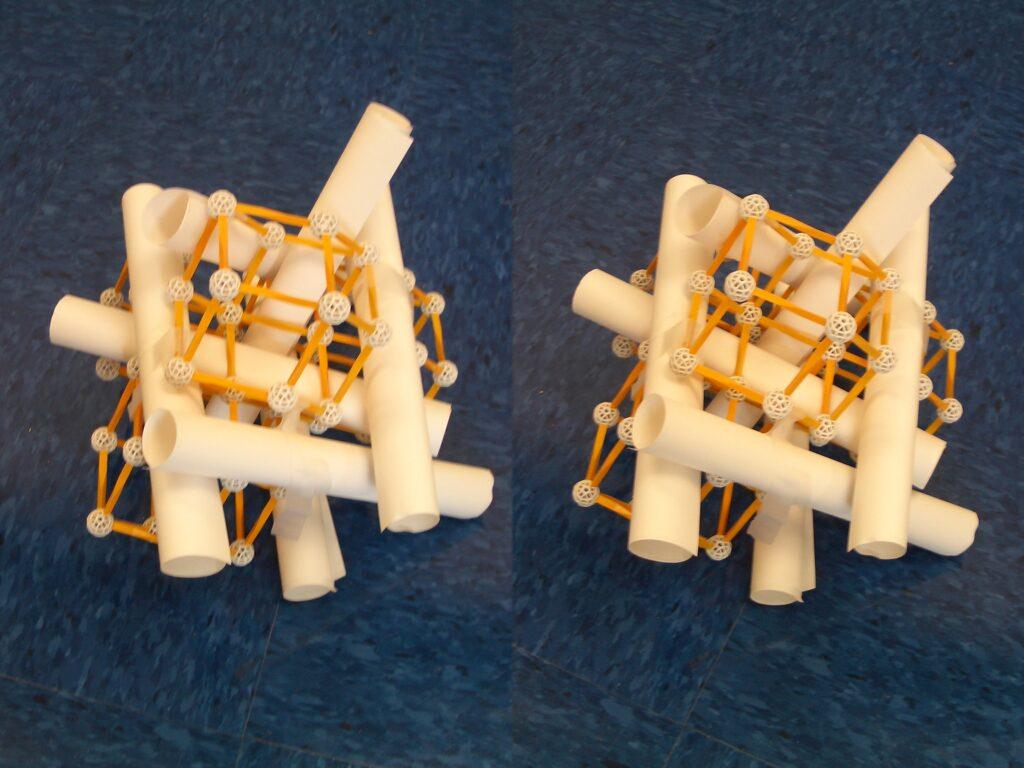
Here are some older experiments:

tetrastix:
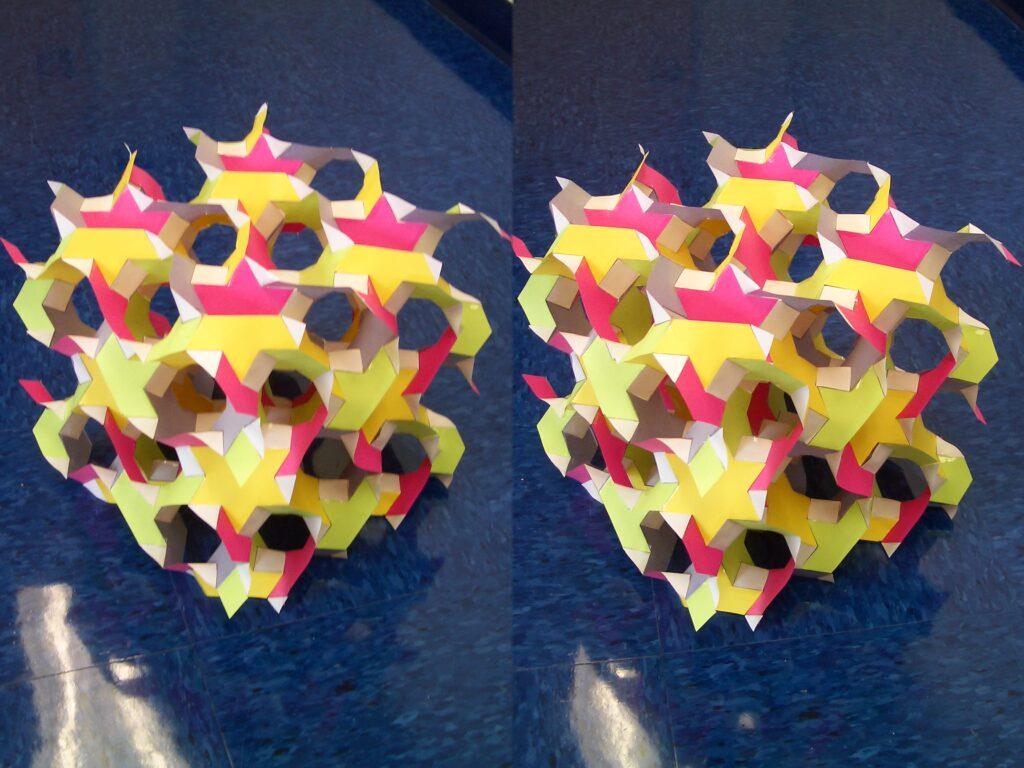
The mu-snub cube, a form that relaxes to the gyroid: