The Magic Theorem
Now appearing, The Magic Theorem, a greatly expanded, much abridged edition of The Symmetries of Things, written with John H. Conway and Heidi Burgiel, now available for sale. (And at a great price for the
Category: mathematical illustration
Tubing Sculpture
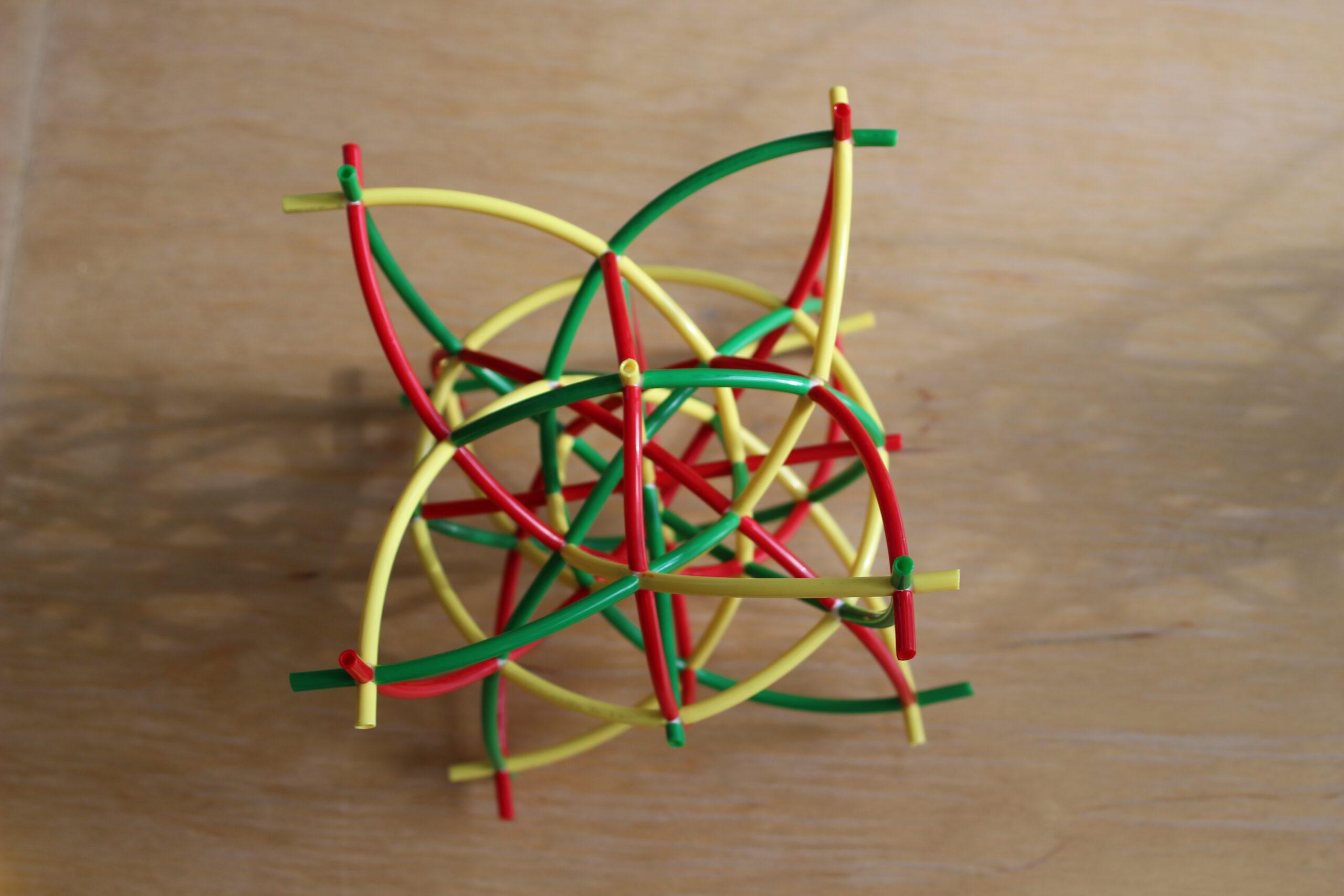
For a few years, I’ve been playing with tubing sculptures, like these, with the aim of illustrating symmetries of the hypersphere, described in the final chapter of The Symmetries of Things. Here are some in
Gyring Gyroid
Gyring Gyroid (w Eugene Sargent, 2012, in honor of Tom Rodgers) This piece of samurai space insect armor shows a particular mathematical surface, the gyroid, that naturally arises in many forms. Discovered by Alan Schoen,
A Woven Klein Quartic
Curvature is determined by local geometry, and this can be controlled. A pattern with a particular symmetry is often in an infinite family of patterns, all with the same underlying motif, but of varied curvature
The Golden Hexagon Hat Tiling Substitution Rules
Inspired by the new substitution rules that Shigeki Akiyama and Yoshiaki Araki propose (along with a new proof of aperiodicity!), here are some drawings from the last few days. These aren’t quite the same as
The Pinwheel Tiling
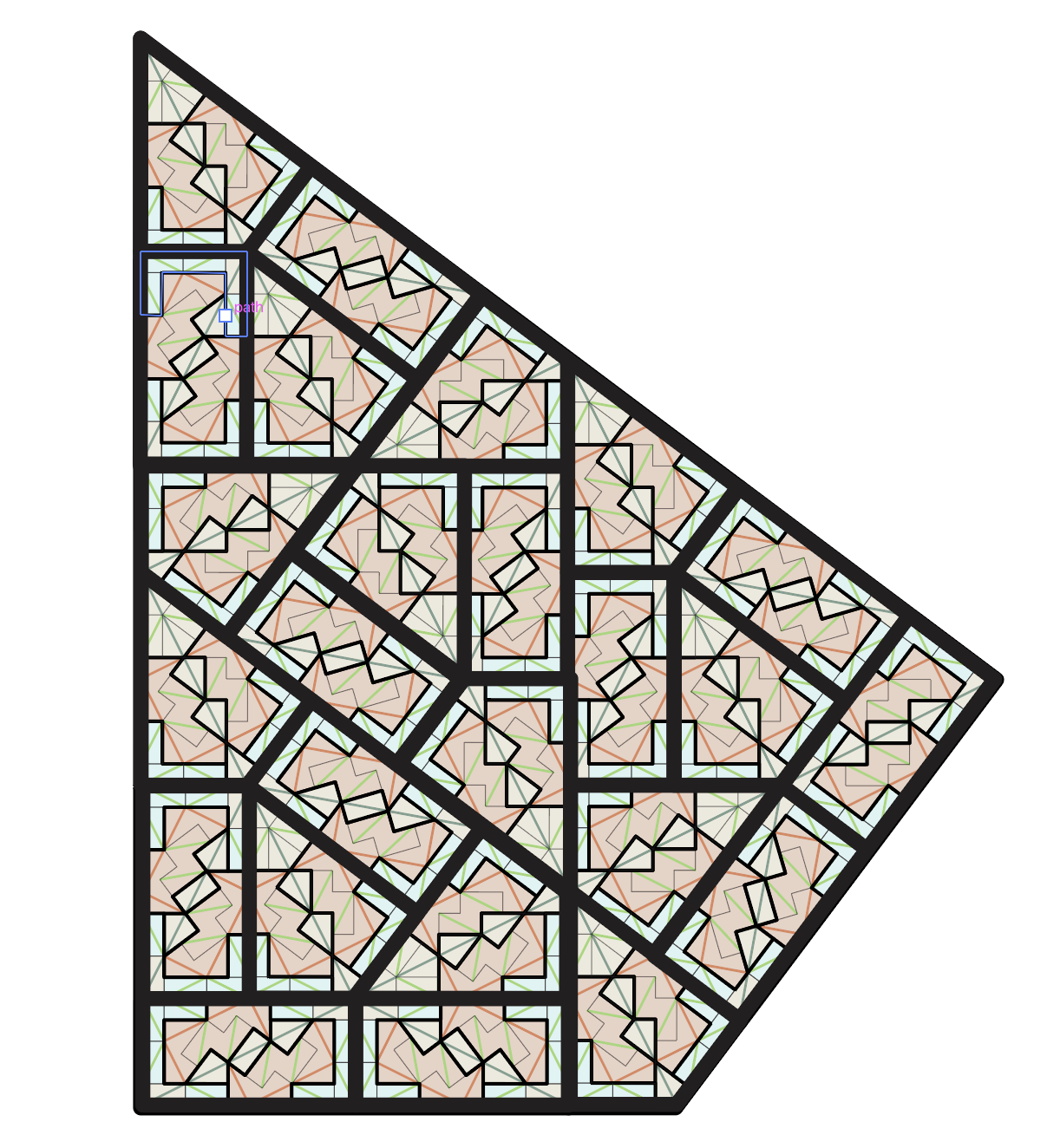
Sometime around 1991, Charles Radin commissioned John Conway for a substitution tiling for which the tiles appeared in infinitely many orientations, and soon John obliged with the Pinwheel. The Pinwheel is formed from right triangles,
Symmetry here and there
All of these photos were taken in 2022, for the upcoming The Magic Theorem of the Symmetries of Things, a second edition of the first part of the book, with expanded exercises and examples, in
A few groovy images
All of these groovy pix use maps from the plane to itself, taking a periodic pattern into the plane in a distorted way. Don’t stare into the middle for too long…
Double Triamond, w/ Hexastix!
“Double Triamond, w/ Hexastix!” created in collaboration with Eugene Sargent, was assembled at the eighth Gathering for Gardner, at Tom Rodgers’ and Sarah Garvin’s beautiful Japanese style gardens and home in Atlanta in 2008. The